Question

How do I find the vertex of `f(x)=x^2-8x+7`?

Answer 1

To find the vertex of `f(x)=x^2−8x+7`, there are several things you can do. You could complete the square to get the equation into vertex form (you can see the vertex in the equation), or the long substitution method . For purposes of understanding, I'll show you the long method.

The first thing you want to do is determine the axis of symmetry (the x co-ordinate of the vertex). To do this, you must factor the equation. In order to factor this simple trinomial, you want to determine 2 numbers that add to give you -8, and multiply to give you +7. These two numbers are -1 and -7:

-7 x -1 = +7
-7 + -1 = -8

Now, you take these two factors and put them in brackets, each with an x like so:

`f(x)=(x - 1)(x - 7)`

Now, set each bracket equal to zero and solve. You should get x = 7 and x = 1. To determine the axis of symmetry, simply add the zeros together and divide by 2:

`(7+1)/2 = 4`

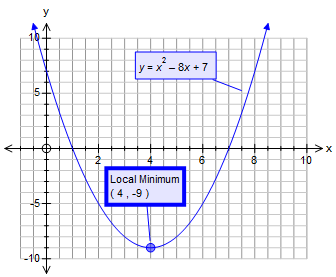
Therefore, the equation for the axis of symmetry is x=4

Now to determine the optimal value (y co-ordinate of the vertex), you can substitute the axis of symmetry into the equation (either the factored form or the original; in this case, factored would me the simplest):

`f(4) = (4-1)(4-7)`

`f(4) = (3)(-3)`

`f(4) = -9` --> Therefore the optimal value is -9.

Putting it together, the vertex is (4, -9)!

Hopefully this was of some help and hopefully you've understood all this! For a faster method, look up "completing the square - vertex form"! :)

Answer 2

Another method!

`P_("vertex")->(x,y)->(4,9)`

Explanation:

Given:`" " y=x^2-8x+7`

`color(blue)("To determine "x_("vertex"))`

This is already in the form `" " y=a(x^2+b/a)+c" as "a=1`

So we can do this 'trick'

Applying `x_("vertex")=(-1/2)(b/a) -> (-1/2)(-8)=+4`

'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
`color(blue)("To determine "y_("vertex"))`

Substitute for `x=(-4)` in the original equation giving:

`" "y_("vertex")=(4)^2-8(4)+7`

`y_("vertex")=+16-32+7= -9`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

`P_("vertex")->(x,y)->(4,9)`