How do I use a graphing calculator to find the roots of the polynomial equation #x^3-4x^2+x+26=0#?
1 Answer
Sep 13, 2014
To find the roots of
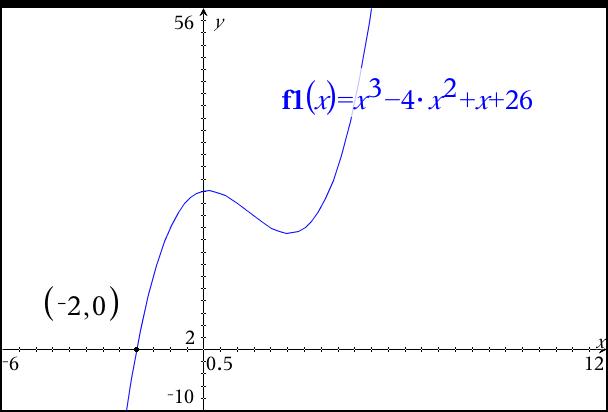
I used a feature called "Analyze" graph to find (-2,0) as the solution.
That is the only real solution to the problem. In order to find the remaining two imaginary/complex solutions, you should perform either long division or synthetic division.
I also used the CAS feature of my calculator to get me the resulting factor:

