Question

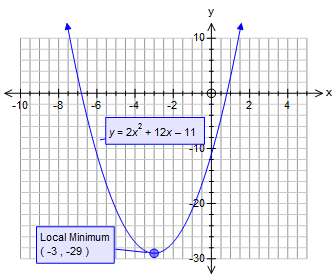
How do you find the axis of symmetry, and the maximum or minimum value of the function `y= 2x^2 + 12x-11`?

Answer 1

Axis of symmetry`" "-> x=-3" "`

Minimum`" "-> (x,y)->(-3,-29)`

Explanation:

The `2x^2` is positive so the general shape of the graph is similar to that of the letter U. Thus we have a minimum

Write as;`" " y=2(x^2+6x)-11`

Consider the `+6" from "6x" "`in the brackets

Multiply this by negative half:

`(-1/2)xx6=-3`

This turns out to be `x_("vertex")=-3`

So the `color(blue)("axis of symmetry is at "x=-3)`, which is line parallel to the y-axis but passes through `x=-3`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
This is also the x-value for the minimum

So by substituting it back into the original equation we find the corresponding value for y

`color(blue)(y_("minimum")=2(-3)^2+12(-3)-11" "=" "-29)`