Question

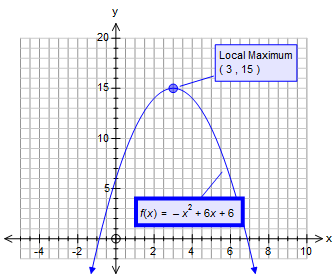
How do you find the axis of symmetry, and the maximum or minimum value of the function `f(x)=-x^2+6x+6`?

Answer 1

Complete the square

The line `x=3` is a line of symmetry

The maximum is `f(3)=15`.

Explanation:

Completing the square gives

`f(x) = -(x-3)^2 + 15`.

Notice that

`f(3+a) = f(3-a)`

for any `a in RR`.

Therefore, the line `x=3` is a line of symmetry.

The maximum is `f(3)=15`.

This is because

`f(3)=15>=15-(x-3)^2=f(x)`

Answer 2

Axis of symmetry is `x=3`.

The maximum`" "->(x,y)->(3,15)`

Explanation:

As the coefficient for `x^2" is "(-1)` the shape type of the quadratic is `nn` so it is a maximum.

Finding axis of symmetry: Let us look at the part of the equation that is `+6x`

Compere to the standard form `y=ax^2+bx+c`

Write standard form as: `y=a(x^2+b/ax)+c`

From this `" "x_("vertex")=(-1/2)xxb/a`

So in your case, as `" "x_("vertex")=(-1/2)xx6`

But `a=(-1)" "`so in fact what you have is:

`" "x_("vertex")=(-1/2)xx6/(-1)" "=" "+3`

Now it is just a matter of substituting `x=3` into the original equation to find that `y=15`