How do you find the axis of symmetry, and the maximum or minimum value of the function #y = x^2 + 3x #?
2 Answers
Of form that has a minimum duo to the coefficient of
Axis of symmetry is:
Explanation:
The
Just for a moment, suppose we had
Consider the general case of
Write this as:
The axis of symmetry is at
So in this case we have:
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Set
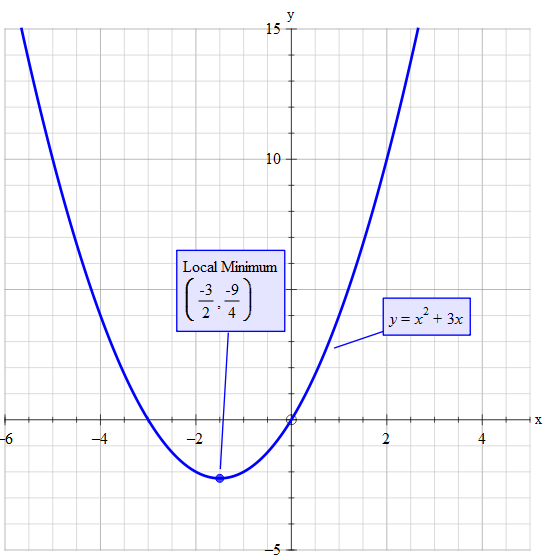
Axis of symmetry
Minimum value
Maximum value
Explanation:
To put the equation in standard form, x-terms are made into a perfect square. In the given expression consider the coefficient of x, which is 3. Half of this is
The equation in this form represents a vertical parabola, opening up, with vertex at
The axis of symmetry is
Minimum value is

