How do you find the axis of symmetry, graph and find the maximum or minimum value of the function #y=-x^2+3x-3#?
1 Answer
Axis of symmetry
Explanation:
As this is in the form of
the axis of symmetry is at
So axis of symmetry is at
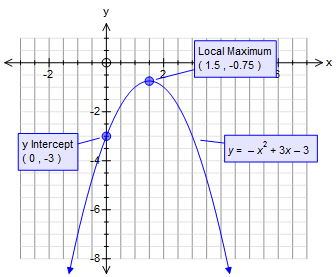
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
As the
We already know that
So the point
As P is a maximum and below the x-axis then we also know that that it does not cross the x-axis. So there is no solution to
To emphasise the point:
In the solution equation:
The part:
giving:
As we are dealing with the sqrt of a negative number this is a sure sign that the graph does not cross the x-axis.

