Question

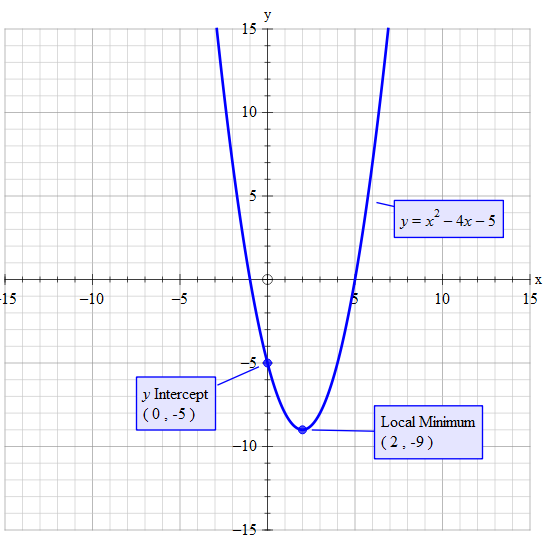
How do you find the y intercept, axis of symmetry and the vertex to graph the function `f(x)=x^2-4x-5`?

Answer 1

See explanation

Explanation:

Using a shortcut trick the use of which depends on the structure of the quadratic.

Consider the standard form of `ax^2+bx+c=y`

Write as `a(x^2+b/ax)+c=y`

Then the y-intercept is `c`
`x_("vertex")=(-1/2)xxb/a larr" watch the signs of "b/a`
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Given: `x^2-4x-5`

`=>y_("intercept")->(x,y)=(0,-5)`

`=>x_("vertex")=(-1/2)xx(-4) = +2`

Now all you need to do is substitute for `x` to determine `y_("vertex")`
I will let you do that!