Question

How do you find the y intercept for `y = 12x^2 - 35x + 8`?

Answer 1

The `y`-intercept is `(0, 8)`.

Explanation:

`y`-intercept is the value at which `x` is zero , or the value of `y` which the point on the line of the graph crosses the `y` axis .

Since `x` has to be `0`, let's substitute `0` for `x` in the equation:
`y = 12x^2 - 35x + 8`
`y = 12(0)^2 - 35(0) + 8`
`y = 0 - 0 + 8`
`y = 8`

Finally, since a `y`-intercept is a point, we must write it as one. So the point is `(0, 8)`.

If we are to graph this, we can see that the graph does indeed have a `y`-intercept of `(0, 8)`:

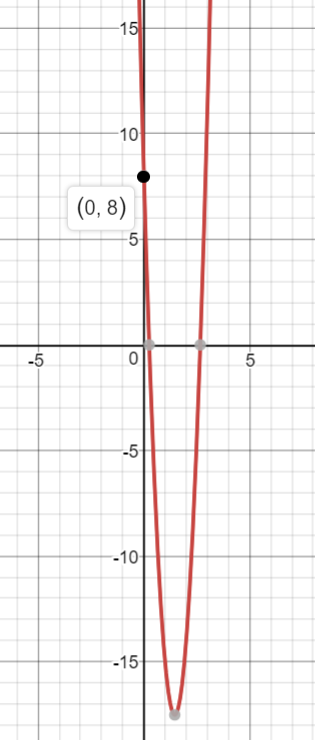
Hope this helps!