How do you FOIL #(3x^2-6)(x^3-9)#?

1 Answer
Meave60
May 31, 2015

#(3x^2-6)(x^3-9)=3x^5-27x^2-6x^3+54#

Problem: FOIL #(3x^2-6)(x^3-9)# .

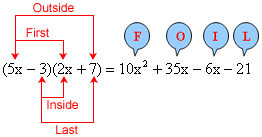
FOIL stands for:

First: Multiply the first term in each binomial together.
Outer/Outside: Multiply the outer/outside term in each binomial together.
Inner/Inside: Multiply the inner/inside term in each binomial together.
Last: Multiply the last term in each binomial together.

http://www.mesacc.edu/~scotz47781/mat120/notes/polynomials/foil_method/foil_method.html
#(3x^2-6)(x^3-9)# =

#(3x^2*x^3)+(3x^2*-9)+(-6*x^3)+(-6*(-9))# =

#3x^5-27x^2-6x^3+54#

Related questions
See all questions in Multiplication of Polynomials by Binomials
Impact of this question
1712 views around the world
You can reuse this answer
Creative Commons License