How do you graph #y=2abs(x+6)-10#?
2 Answers
Feb 12, 2017
See the explanation
Explanation:
The bit inside the | | can be positive or negative but writing it like
At
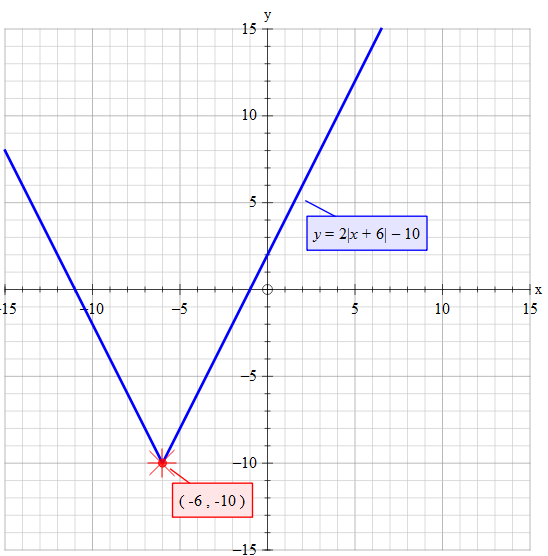
Feb 12, 2017
See the Socratic graph and explanation.
Explanation:
So,
The equation represents the point of intersection #V(-6, -10) and the
V-part above, of the pair of lines
Algebraic proof:
In addition to
The Socratic graph is inserted.
graph{2|x+6|-10 [-20, 20, -11, 9]}

