How do you solve -6x+14<-28 or 9x+15<-12?

Question

14666 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-06-20T01:47:24

#(-oo,-3) uu (7,oo)#

Solve each inequality separately, then combine them with the "or" operator, and then simplify if possible.

Let's start with the first inequality:

#-6x+14 < -28#

#-6x < -42#

#6x > 42#

#x > 7#

Now, we solve the second inequality:

#9x+15 < -12#

#9x < -27#

#x < -3#

Combining the two, we get:

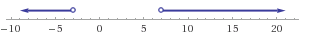
#x < -3 or x > 7#

This cannot be simplified, since the two solution regions do not overlap. The more formal way to write this (with interval notation) is:

#(-oo,-3) uu (7,oo)#

On a number line, the solution set looks like this:

Final Answer