sente gave the solution #x in(-oo,-2)#
It appears that there are several conventions regarding notation:
If #x < -2# then it takes on all values less than -2 and thus #underline("excluding -2")#.
So by using the square bracket notation it would be written as:
" #x in[-oo , -2color(white)(.)[color(white)(...)#"..... where the reversed square bracket # [ -> " not inclusive"#
#color(red)("However, it appears that this is not correct")#
From the link:
https://en.wikipedia.org/wiki/Interval_%28mathematics%29#Including_or_excluding_endpoints
We have:
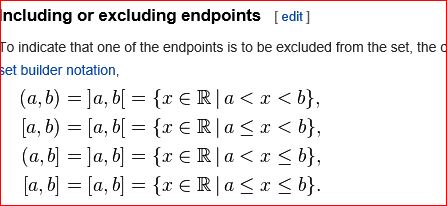
The presented solution of #x in (-oo,-2)# when compared to first line given in Wikipedia gives cause for some debate.
The Wikipedia table #color(red)(underline("implies"))# that #x in (oo,-2)# is such that #x < -2 # which is absolutely true but unfortunately it also implies that #oo < x # which is not true.
However I have been given some guidance by a couple of very informed Mathematicians that the
#color(green)("the use of curved brackets is indeed correct for this context.")#
#color(red)("By some conventions that I was not previously aware of")#
#color(red)("it would not be correct to write")#
#color(red)([-oo ,-2) -> {x in RR | -oo<=x<-2})#.
I am advised that this is because the use of [ is only applicable if #x in RR#. The problem is that #oo notin RR# so the use of [ is not permitted.

