Question

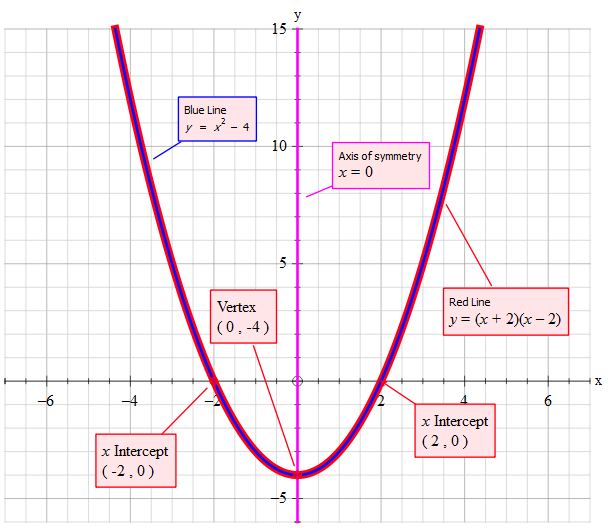
How do you tell whether the graph opens up or down, find the vertex, and find the axis of symmetry of `y=(x+2)(x-2)`?

Answer 1

Seee explanation.

Explanation:

If a quadratic function is given in such form then:

1. Its zeroes are `2` and `-2`
2. The axis of symetry can be calculated as the mean of the zeros:
   `p=(x_1+x_2)/2=0`, so the axis is `x=0`
3. The vertex can be calculated as `q=f(0)=(0+2)(0-2)=-4`, so `V=(0,-4)`
4. If the graph is given in such form then to check if it opens up or down you check if the expressions in brackets are multiplied by a positive or negative constant.

If the constant is positice (as here) the graph opens up, if it is negative, the graph opens down.

Answer 2

Opens up

Axis of symmetry is `x=0`

Vertex`->(x,y)=(0,-4)`

Explanation:

If you multiply out the brackets the first term is `x^2`

The number in front (not shown) is +1

So as the `x^2` is positive the graph is of general shape `uu`
Using your words: it opens up

The whole thing multiplied out is `y=x^2-4`

~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

The given for of `y=(x+2)(x-2)` is useful as it may be used to determine where the graph crosses the x-axis. The axis of symmetry passes through the mid point.

Set `" "y=0=(x+2)(x-2)`

There are 2 condition for `y=0`

condition 1: `(x+2)=0 => x=-2`
condition 2: `(x-2)=0 => x=+2`

The mid point is: `" "x=(+2-2)/2=0/2=0`

So the axis of symmetry is `x=0`
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
The `x_("vertex")` has the same value as the axis of symmetry.

`x_("vertex")=0`

To find `y` substitute 0 for `x`

`y=(x+2)(x-2)" "->" "y=(0+2)(0-2) = -4`

Vertex`->(x,y)=(0,-4)`