How do you write the parabola #2y^2+4y+x-8=0# in standard form and find the vertex, focus, and directrix?

2 Answers
Tony
Jun 1, 2018

You try to make a perfect square!

Explanation:

#2(y^2 +2y +1 -1) +x -8 = 0#

#2(y+1)^2-2+x-8=0#

#2(y+1)^2+x-10=0#

#2(y+1)^2 = -(x-10)#

#(y+1)^2 = -1/2(x-10)#

#(y+1)^2 = 4(-1/8)(x-10)#

Douglas K.
Jun 1, 2018

This is a parabola that opens to the left, therefore, its standard form is:

#x = ay^2+by+c , a < 0#

The formulas for the requested items will be given in the explanation.

Explanation:

Given: #2y^2+4y+x-8=0#

To write in standard form, we add #-2y^2-4y+8# to both sides:

#x = -2y^2-4y+8#

The above is standard form where #a = -2#, #b = -4#, and #c = 8#

The vertex is a point #(h,k)#

The formula for the y-coordinate of the vertex is:

#k = -b/(2a)#

Substitute in the values, #a = -2# and #b = -4#

#k = -(-4)/(2(-2)#

#k = -1#

The formula for the x-coordinate of the vertex is:

#h = ak^2+b(k) + c#

Substitute in the values, #h = -1#, #a = -2#, #b = -4#, and #c = 8#

#h = -2(-1)^2-4(-1) + 8#

#h =10#

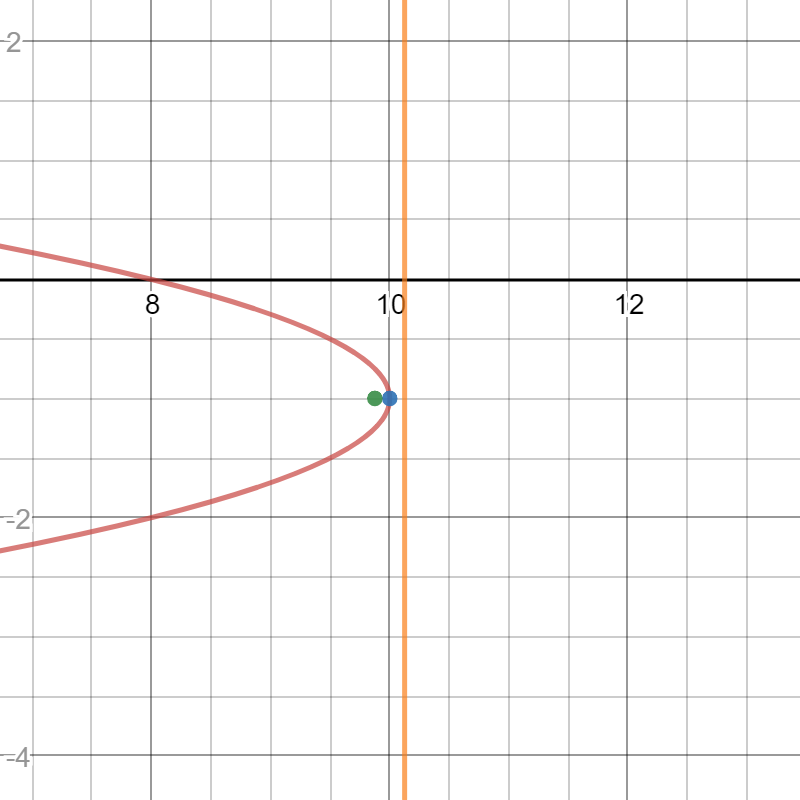
The vertex is #(10,-1)#

The focal distance #f# is:

#f = 1/(4a)#

#f = 1/(4(-2))#

#f = -1/8#

The formula for the focus is:

#(h+f, k)#

Substitute in values #h = 10#, #f = -1/8#, and #k = -1#

#(10-1/8, 10)#

The focus is #(79/8, -1)#

The formula for the equation of the directrix is:

#x = h-f#

Substitute in values #h = 10# and #f = -1/8#:

#x = 10 - -1/8#

#x = 81/8#

The above is the equation of the directrix.

The following is a drawing of the parabola, the vertex, the focus, and the directrix:

www.desmos.com/calculator

Related questions
See all questions in Identify Critical Points
Impact of this question
6546 views around the world
You can reuse this answer
Creative Commons License