How many half-lives will it take to reach 6.25% of its original concentration?

Question

If A(g) #-># B(g) has k=#2.3*10^-3# #s^-1#, how many half-lives will it take for the concentration of A to reach 6.25% of its original concentration?

28626 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-03-28T02:41:04

It will take 4 half lives.

1st gets you to 50%
2nd gets you to 25%
3rd gets you to 12.5%
4th gets you to 6.25%

#(1/2)^n, n = 4; (1/16)xx100 = 6.25%#

Answer 2 · 2018-03-28T02:41:09

#"4 half lives"#

#"A"_"(g)" -> "B"_"(g)" color(white)(...)"k" = 2.3 × 10^-3\ "s"^-1#

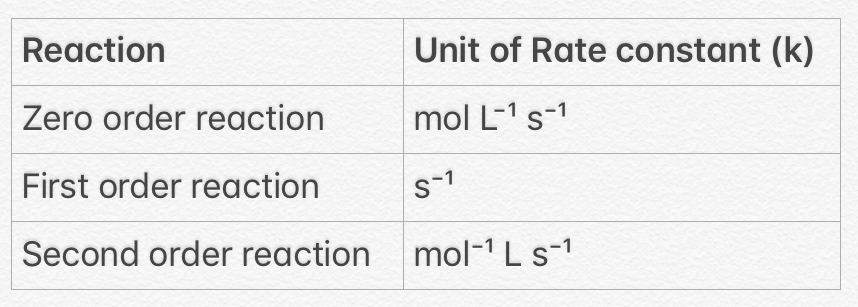
Here, unit of Rate constant #("k")# is #"s"^-1#. Therefore, it’s a first order reaction

Units of Rate constant in zero, first and second order reactions.
enter image source here

For a first order reaction, we can write

#"N" = "N"_0/2^"n"#

Where

#"N"_0 =# Initial amount of substance

#"N ="# Amount of substance after #"n"# half lives

#"n ="# Number of half lives

#6.25% = (100%)/2^"n"#

#2^"n" = (100%)/(6.25%)#

#2^"n" = 16#

#2^"n" = 2^4#

#"n" = 4#

∴ It takes #4# half lives for concentration of #"A"# to reach #6.25%#