If the ratio of the sides of two similar triangles is 4:9, how do you find the ratio of their areas?
2 Answers
Explanation:
Scale factor for the sides of these triangles.
Therefore the ratio of area will be:
Ratio of their areas is
Explanation:
Let us have two similar triangles
Let us also draw perpendiculars
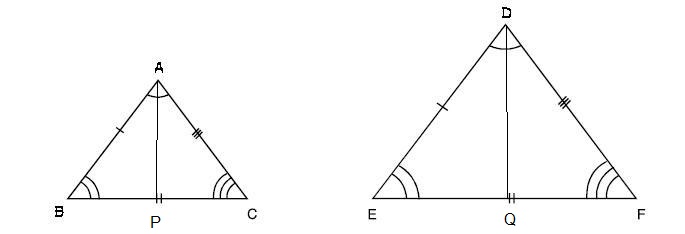
It is apparent that
We also have
But
Hence if sides of two similar triangles are in the ratio
As in given case sides are in the ratio of
ratio of their areas is


