In an isosceles triangle, the vertex angle is twice the each base angle. What is the vertex angle?
2 Answers
Nov 14, 2015
Explanation:
Since the sum of the interior angles of any triangle adds up to
Therefore the vertex angle is
Jan 9, 2018
Explanation:
An isosceles triangle has two sides of equal length. Likewise, the opposite angles of the sides are the same
So lets assume each angle be
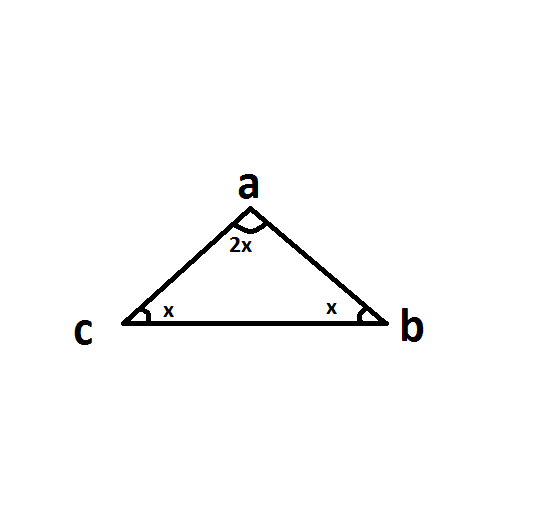
Now, we know that sum of the angles in a triangle is 180 degree
So,
Now the other angle is
Hope this helps!!! ☺☻ have a good day...

