Let R be the shaded region in the first quadrant enclosed by the y-axis and the graphs of #y=4-x^2# and #y=1+2sinx#, how do you find the volume of the solid whose base is R and whose cross sections perpendicular to the x-axis are squares?
1 Answer
Jul 12, 2016
Explanation:
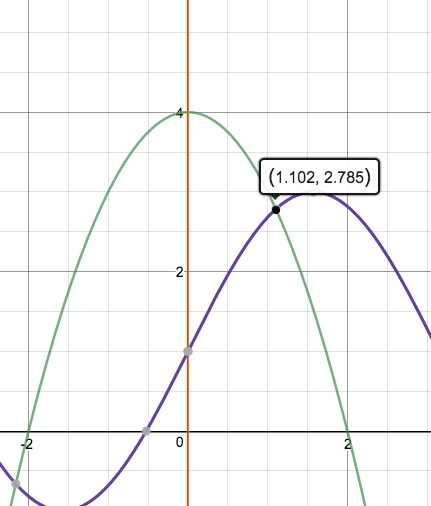
at any given x, the cross section area of the square will be
with
and
so volume V(x) follows as
Please note that I got the 1.102 off the Desmos plot included here. I did not solve it myself.
Also that integral is horrendous so I would recommend a computer solution if you have access. This is really not something you want to be doing by hand.

