Question

Two opposite sides of a parallelogram each have a length of `4`. If one corner of the parallelogram has an angle of `(5 pi)/6` and the parallelogram's area is `56`, how long are the other two sides?

Answer 1

The other length is 28

Explanation:

Notice that the wording of the question is such that we can chose which of the opposite sides we may assign the length of 4.

`color(blue)("Declaring all the relationships")`

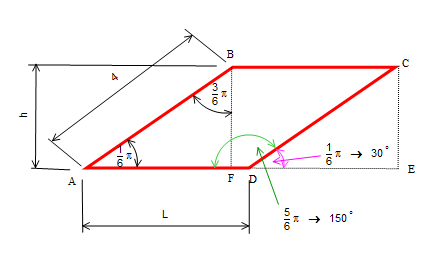
Given than `angle ADC = 5/6pi =>angle EDC=angle DAB=1/6pi->30^o`

Let `angle AFB = pi/2`

Then `Delta AFB` is half of an equilateral triangle

Thus `angle ABF = 3/6pi`

Assign the length of 4 to AB

Let the length `AD = L`

Given that the area =56

Known: area`=hL` where `h->FB`

'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
`color(blue)(" Calculating the other length")`

Thus we have: `area=Lh=56`

Known: `h=4sin(1/6pi)`

`=>Lxx4sin(1/6pi)=56`

But `sin(1/6pi)=1/2` giving

`" "(4L)/2=56`

`color(blue)(=>L=(2xx56)/4 =28 )`
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~