Question

Two rhombuses have sides with lengths of `9`. If one rhombus has a corner with an angle of `(3pi)/8` and the other has a corner with an angle of `(7pi)/12`, what is the difference between the areas of the rhombuses?

Answer 1

Difference in areas of the Rhombuses 3.4056

Explanation:

Area of rhombus `= (1/2) * d_1 * d_2 or a * h`
Where `d_1 , d_2` are the diagonals, a is the side and h is the altitude.

In this case we will use the formula Area = a * h.

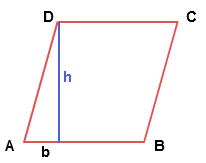
Rhombus 1
`h = a sin theta = 9 * sin ((3pi)/8) = 8.3149`
Area = a * h = 9 * 8.3149 = 74.8341#

Rhombus 2
`h = a sin theta = 9 * sin ((7pi)/12) = 8.6933`
Area = a * h = 9 * 8.6933 = 78.2397

Hence the difference in areas is `78.2397 - 74.8341 = 3.4056`