Question

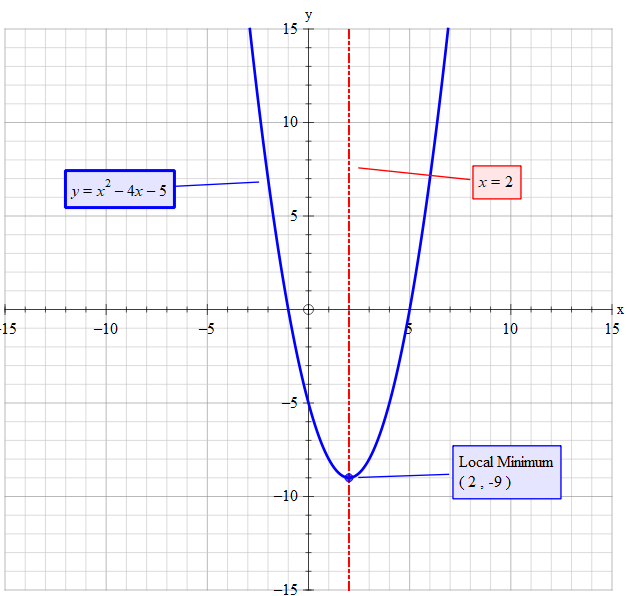
What is the axis of symmetry and vertex for the graph `F(x) = x ^ 2 - 4x - 5`?

Answer 1

This is not a conventional way to derive the answer. It uses part of the process for 'completing the square'.

Vertex `->(x,y)=(2,-9)`
Axis of symmetry `->x=2`

Explanation:

Consider the standard form of `y=ax^2+bx+c`

Write as :`y=a(x^2+b/a x) +c`

`x_("vertex")="axis of symmetry" = (-1/2)xxb/a`

The the context of this question `a=1`

`x_("vertex")="axis of symmetry" = (-1/2)xx(-4)/1 = +2`

So by substitution

`y_("vertex")=(2)^2-4(2)-5 = -9`

Thus we have:

Vertex `->(x,y)=(2,-9)`
Axis of symmetry `->x=2`