Question

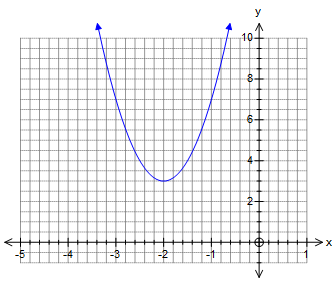
What is the vertex of `y=4(x + 2)^2 + 3`?

Answer 1

Vertex`->(x,y)->(-2,3)`

Explanation:

Consider the `color(blue)(2)` in `(x+ color(blue)(2))`

`x_("vertex")= (-1)xx color(blue)(2)=color(red)(-2)`

Now that you now the value for `x` all you need to do is substitute it back into the original formula to obtain the value of y

So `y_("vertex")=4((color(red)(-2))+2)^2+3`

`y_("vertex")=3`
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
The equation form of `y=4(x+2)^2+3` is also known as completing the square. It is derived from the standard quadratic form of
`y=ax^2+bx+c`

For this question its standard quadratic form is:
`y=4(x^2+4x+4)+3`
`y=4x^2+16x+19`
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~