Why can't you prove triangles congruent by using side-side-angle?
1 Answer
Jan 6, 2016
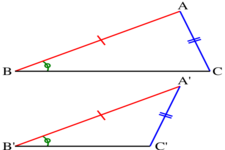
Consider the two triangles.
The red sides are congruent, the blue sides are congruent, and the green angles are congruent.
However, even though the triangles would seem to be side-side-angle congruent, they're not. Every side-side-angle combination can be used to describe two different triangles.
(The one exception is if the angle is a right angle, for which only one triangle can be drawn. This special case of side-side-angle congruence is called the hypotenuse leg theorem and is valid.)

