Question

How do you graph `x + y < 3` and `x – y < 4`?

Answer 1

Find the intercepts and asymptotes, plot a few points, and then sketch the graph.

Explanation:

`y=40^x`

Step 1. Find the domain and range.

`y` is defined for all real values of `x`, so the domain is the set of all real numbers.

`40^x>0`, so the range is `y>0`.

Step 2. Find the `y`-intercept.

Let `x=0`.

`y= 40^0 = 1`

The `y`-intercept is at (`0,1`).

Step 3. Find the `x`-intercept.

There is no `x`-intercept, because the domain is `y>0`.

Step 4. Find the horizontal asymptote.

As `x` decreases without bound, `40^x` approaches `0`.

The horizontal asymptote is at `y=0`.

Step 5. Calculate some extra points.

We have one point: `f(0) =1`.

`f(1/2) =40^(1/2) = sqrt40 ≈ 6.3`

`f(-1/2) = 40^(-1/2) = 1/sqrt40 ≈0.16`

Step 6. Plot the axes and your three points.

Step 7. Complete the graph with a smooth curve through the three points.

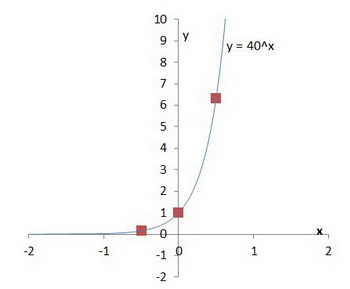
And you have your graph.