How do you multiply polynomials #(7x^3 - 6x^2 + 4x-8) (9x^3 - 2x^2 - 3x +5) #?
2 Answers
Collect the coefficients of the product using a grid to find:
#(7x^3-6x^2+4x-8)(9x^3-2x^2-3x+5)#
#=63x^6-68x^5+27x^4-27x^3-26x^2+44x-40#
Explanation:
One way is to write out a grid of the coefficients and their products as follows:
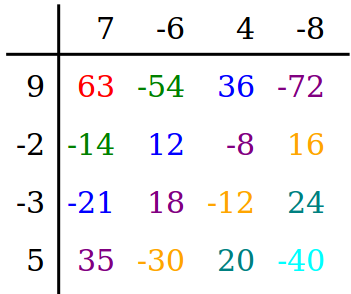
Each reverse diagonal corresponds to choices of terms from each of the two original polynomials that result in the same power of
Sum the reverse diagonals to get the coefficients of the product of the polynomials:
#(7x^3-6x^2+4x-8)(9x^3-2x^2-3x+5)#
#=color(red)(63)x^6+(color(green)(-54-14))x^5+(color(blue)(36+12-21))x^4+(color(purple)(-72-8+18+35))x^3+(color(orange)(16-12-30))x^2+(color(teal)(24+20))x+color(cyan)(-40)#
#=color(red)(63)x^6-color(green)(68)x^5+color(blue)(27)x^4-color(purple)(27)x^3-color(orange)(26)x^2+color(teal)(44)x-color(cyan)(40)#
Explanation:
#{: (" X ", " | ", 9x^3,-2x^2,-3x,+5), ("-----","-+-","-------","-------","-------","--------"), (7x^3," | ",color(orange)(63x^6),color(red)(-14x^5),color(blue)(-21x^4),color(green)(35x^3)), (-6x^2," | ",color(red)(-54x^5),color(blue)(12x^4),color(green)(18x^3),color(brown)(-30x^2)), (+4x," | ",color(blue)(36x^4),color(green)(-8x^3),color(brown)(-12x^2),color(purple)(20x)), (-8," | ",color(green)(-72x^3),color(brown)(16x^2),color(purple)(24x),color(cyan)(-40)) :}#

