Question

Where are the `3d_(xy)` orbitals relative to `3d_(z^2)` in an octahedral metal complex?

Answer 1

Well, a transition metal that forms an octahedral complex (hence, coordination number = `6`), such as `["Mn""O"_6]^(2+)`, would have its ligands lying on/along the x, y, and z axes, not in between them. However:

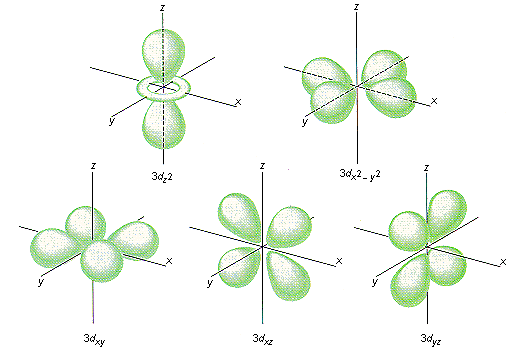
You can also see that the `3d_(xy)` orbitals have their lobes in between the x and y axes, which is less spatially obstructive to the equatorial ligands.

On the other hand, the `3d_(z^2)` orbitals have two lobes lying directly on/along the z-axis, in-line with the axial ligands, creating spatial obstruction and increasing their energy.

You can further expect that since the metal is positively charged, the ligands tend to have negative charges, hence they possess electrons in their own orbitals that can repel the electrons in the transition metal's orbitals.

So, I would expect the `\mathbf(3d_(xy))` orbitals to be lower in energy.

And in fact, they are, because an octahedral complex has a crystal field splitting like this:

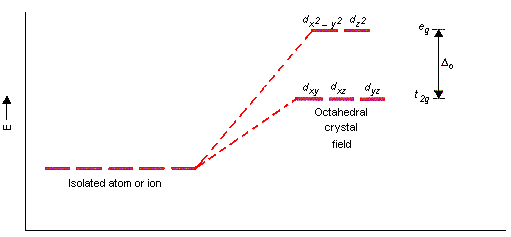
where `Delta"o"` is the crystal field splitting energy, `e_g` is the 'class' of orbitals higher in energy, and `t_(2g)` is the 'class' of orbitals lower in energy.