Question

How do you find the volume of the solid formed by rotating the region enclosed by ?

about the x-axis.

Answer 1

`V=pi(e^(0.6)/2+4e^0.3-3.3)~~9.4577...`

Explanation:

We'll use a method call the disk method to do this.

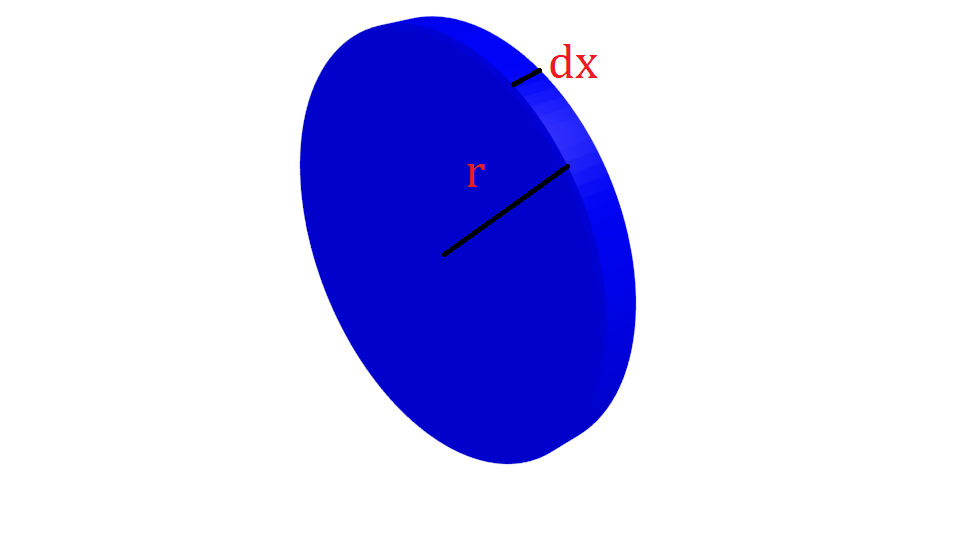
Take a look at the above picture. Pretend it's an infinitely small cylindrical disk, with radius `r` and height `dx`. When we revolve `y=e^x+2` about the `x`-axis, we can break the resulting solid down to infinitely many infinitely small cylindrical disks like the one above (woah, calculus huh!). All of these disks have a radius equal to the value of the `y` and height equal to an infinitely small change in `x`, or `dx`.

We know volume of a cylinder is given by:
`V=pir^2h`
And we know that the radius of each cylinder is the value of `y`, and the height is `dx`; that means the volume of an infinitely small disk is:
`dV=pi(y^2)dx`
Integrating both sides to find total volume, for `x=0` to `x=0.3`, gives
`V=int_0^0.3pi(y^2)dx`
But `pi` is a constant and `y=e^x+2`, so
`V=piint_0^0.3(e^x+2)^2dx`

Expanding the `(e^x+2)^2` binomial:
`V=piint_0^0.3e^(2x)+4e^x+4dx`
And using the sum rule:
`V=piint_0^0.3e^(2x)dx+piint_0^0.3 4e^xdx+piint_0^0.3 4dx`
Finally, evaluating these one by one:
`V=pi[e^(2x)/2]_0^0.3+4pi[e^x]_0^0.3+4pi[x]_0^0.3`
`V=pi((e^(0.6)/2-e^0/2)+4(e^0.3-e^0)+4(0.3-0))`
`V=pi(e^(0.6)/2-1/2+4e^0.3-4+1.2)`
`V=pi(e^(0.6)/2+4e^0.3-3.3)~~9.4577...`

Answer 2

Awesome Ken...

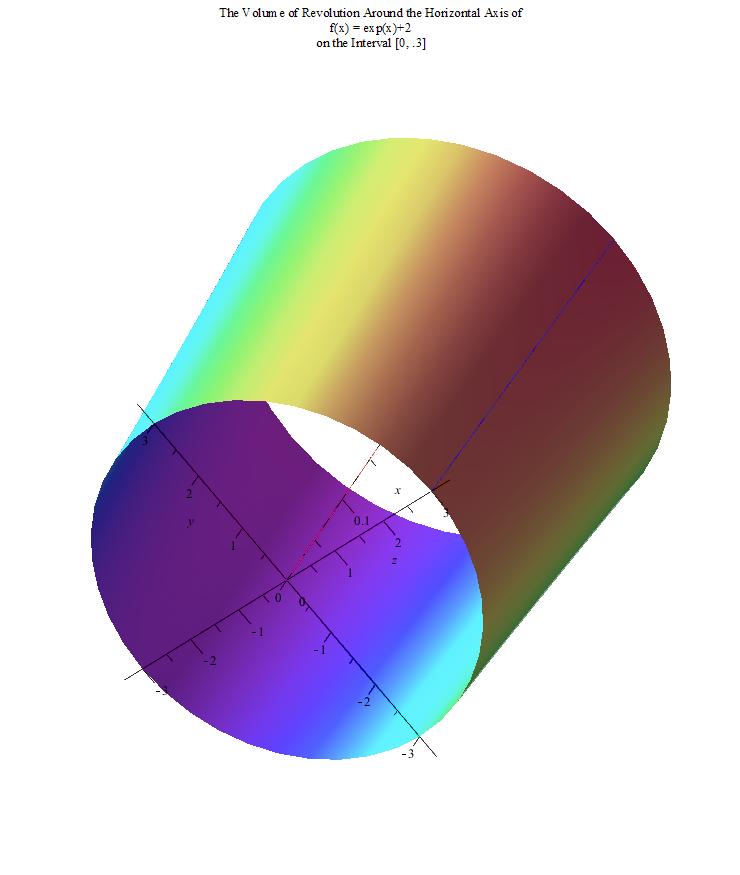
Here is a Maple rendering of the rotation!

By the way, you have the coolest name in the world.

Answer 3

0.9117

Explanation:

`f(r)=e^x`
`V(R)=2 pi int_0^R f(r)rdr`
`C(h,r)=pi h r^2` now with `h = 2` and `R = 0.3`
`V_t =V(R) + C(2,R) = 0.3462+0.5655 = 0.9117`