A triangle has corners at points A, B, and C. Side AB has a length of #33 #. The distance between the intersection of point A's angle bisector with side BC and point B is #15 #. If side AC has a length of #27 #, what is the length of side BC?

Question

1256 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-06-06T09:18:40

#BC=27 3/11# units

Please refer to figure below.
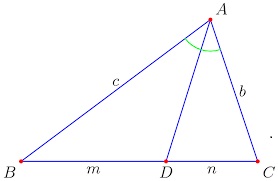
enter image source here

Here let #a=BC#,
#b=AC=27# and
#c=AB=33#.

Further, bisector of angle #A# cuts #AB# at #D# and #BD=15#

In such a triangle according to angle bisector theorem, bisector of angle #A#, divides #BC#

in the ratio of the two sides containing the angle.

In other words, #(AB)/(AC)=(BD)/(DC)# and hence here we have

#33/27=15/(DC)# or #33xxDC=27xx15# and

#DC=(27xx15)/33=(9cancel(27)xx15)/(11cancel(33))=135/11=12 3/11#

Hence #BC=15+12 3/11=27 3/11#