Question

How do you find vertical, horizontal and oblique asymptotes for `(x^2 - 2x + 3) / x`?

Answer 1

Vertical asymptotes:

Vertical asymptotes occur when the denominator of a rational function equals to 0 (this being because division by 0 is undefined in mathematics). We can find any vertical asymptotes by setting the denominator to 0 and solving.

`x = 0`

`x = 0`

There will be a vertical asymptote at `x = 0`

Horizontal asymptotes:

Horizontal asymptotes only occur when the degree of the denominator is higher or equal to that of the numerator. We don't have this situation in our function.

Oblique asymptotes:

Oblique asymptotes occur when the denominator has a lower degree than the numerator. If the function is `f(x) = (g(x))/(h(x))`, there will be an oblique asymptote at the quotient of `g(x)/ (h(x))`.

Therefore, we will have to divide your rational function. A thorough understanding of division of polynomials is usually a pre-requisite to finding oblique asymptotes.

By synthetic division:

`"0_| 1 -2 3"`
`" 0 0 0"`
`"---------------"`
`" 1 -2 3"`

The quotient is therefore `x - 2`, with the remainder being `3`.

There will therefore be an oblique asymptote at `y = x - 2`

Here is the graph of the function:

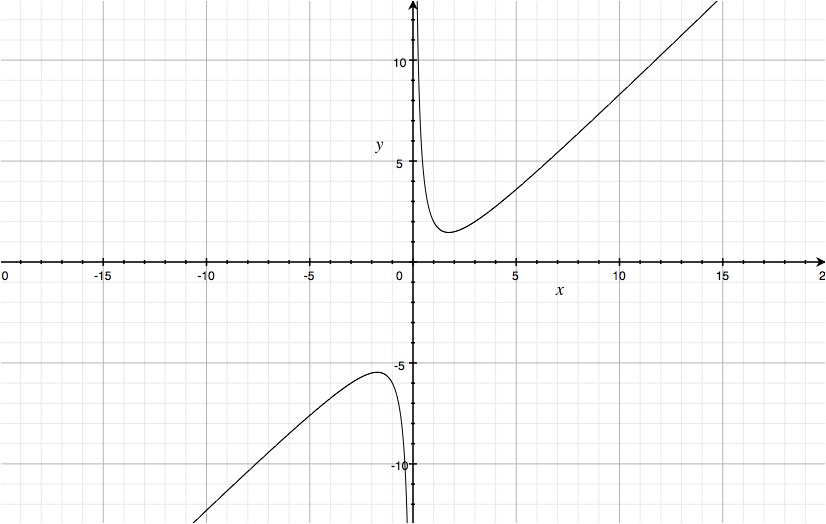
Hopefully this helps!