Question

What are the set of four quantum numbers that represent the electron gained to form the `Br` ion from `Br` atom?

Answer 1

`n = 4, l = 1, m_l = 0, m_s = -1/2`

Explanation:

As you know, there are four quantum numbers used to describe the position and spin of an electron in an atom.

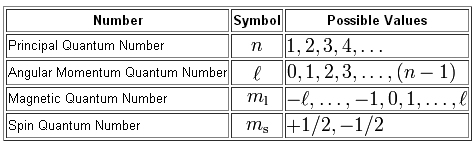
Your goal here will be to use the information provided by the electron configuration of a neutral bromine atom, `"Br"`, to determine the quantum numbers associated with the electron needed to form the bromide anion, `"Br"^(-)`.

So, bromine is located in period 4, group 17 of the periodic table and has an atomic number equal to `35`. This tells you that a neutral bromine atom has a total of `35` electrons surrounding its nucleus.

The electron configuration of a neutral bromine atom looks like this

`"Br: " 1s^2 2s^2 2p^6 3s^2 3p^6 3d^10 color(red)(4) s^2 color(red)(4)p^5`

Notice that bromine's outermost electrons, i.e. its valence electrons, are located on the fourth energy level, `n = color(red)(4)`.

The incoming electron will be added to this energy level, so right from the start you know that it must have `n=4`.

The angular momentum quantum number, `l`, tells you the subshell in which the electron is located. In this case, the incoming electron will be added to the 4p-subshell, which is characterized, much like any p-subshell, by `l=1`.

The magnetic quantum number, `m_l`, tells you the exact orbital in which the electron is located. The 4p-subshell contains a total of `3` p-orbitals

• `4p_x -> m_l = -1`
• `4p_y -> m_l = +1`
• `4p_z -> m_l = color(white)(-)0`

This is used by convention because the wave function associated with `m_l = 0` is said to be symmetric about its axis, I.e. it has no component of angular momentum about its axis, which is usually chosen as the `z` axis.

Since the neutral bromine atom already has 5 electrons in its 4p-subshell, you can say that its `4p_x` and `4p_y` orbitals are completely filled and the `4p_z` contains one electron.

The incoming electron will thus be added to the half-empty `4p_z` orbital, and so it will have `m_l = 0`.

Finally, the spin quantum number, `m_s`, tells you the spin of the electron. Because the `4p_z` already contained one electron that has spin-up, or `m_s = +1/2`, the incoming electron must have an opposite spin, and so `m_s = -1/2`.

The quantum number set that describes the incoming electron will thus be

`color(green)(|bar(ul(color(white)(a/a)color(black)(n = color(red)(4), l = 1, m_l = 0, m_s = -1/2)color(white)(a/a)|)))`

SIDE NOTE You'll sometimes see this notation used for the magnetic quantum number

• `4p_x -> m_l = -1`
• `4p_y -> m_l = color(white)(-)0`
• `4p_z -> m_l = +1`

You can use this if you want, but make sure that you are consistent.

In this notation, the wave function associated with `m_l = 0` has symmetry about the `y` axis, so make sure that you specify and keep track of this for other orbitals such as the d-orbitals of f-orbitals.