Question

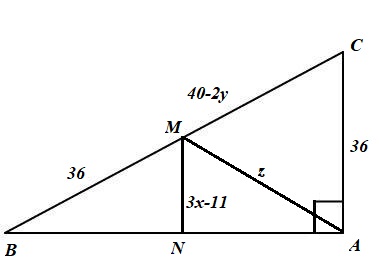
In the `DeltaABC` below, `M` and `N` are midpoints of `BC` and `AB` respectively, `m/_A=90^@`. Find `x,y` and `z`?

Answer 1

`x=9.67`, `y=2` and `z=36`

Explanation:

As M is the midpoint of BC, we have

`36=40-2y` and transposing similar terms we get

`2y=40-36=4`. Hence, `y=2`.

As `M` is the midpoint of `BC` and it joins mid point of `AB` at the base at `N`, then `MN` is half of `AC=36` and hence

`3x-11=36/2=18` i.e.

`3x=18+11=29` and `x=29/3=9.67`.

As `MN` joins mid points of `AB` and `BC`, it too is perpendicular to `AB` (as `MN`||`AC`) and `DeltasMNB` and `AMN` are congruent (SAS) and hence `BM=AM`.

Hence, `z=36`.