Question

What is the half-life of a first-order reaction with a rate constant of `7.80xx10^-4 s^(-1)`?

Answer 1

`"889 s"`

Explanation:

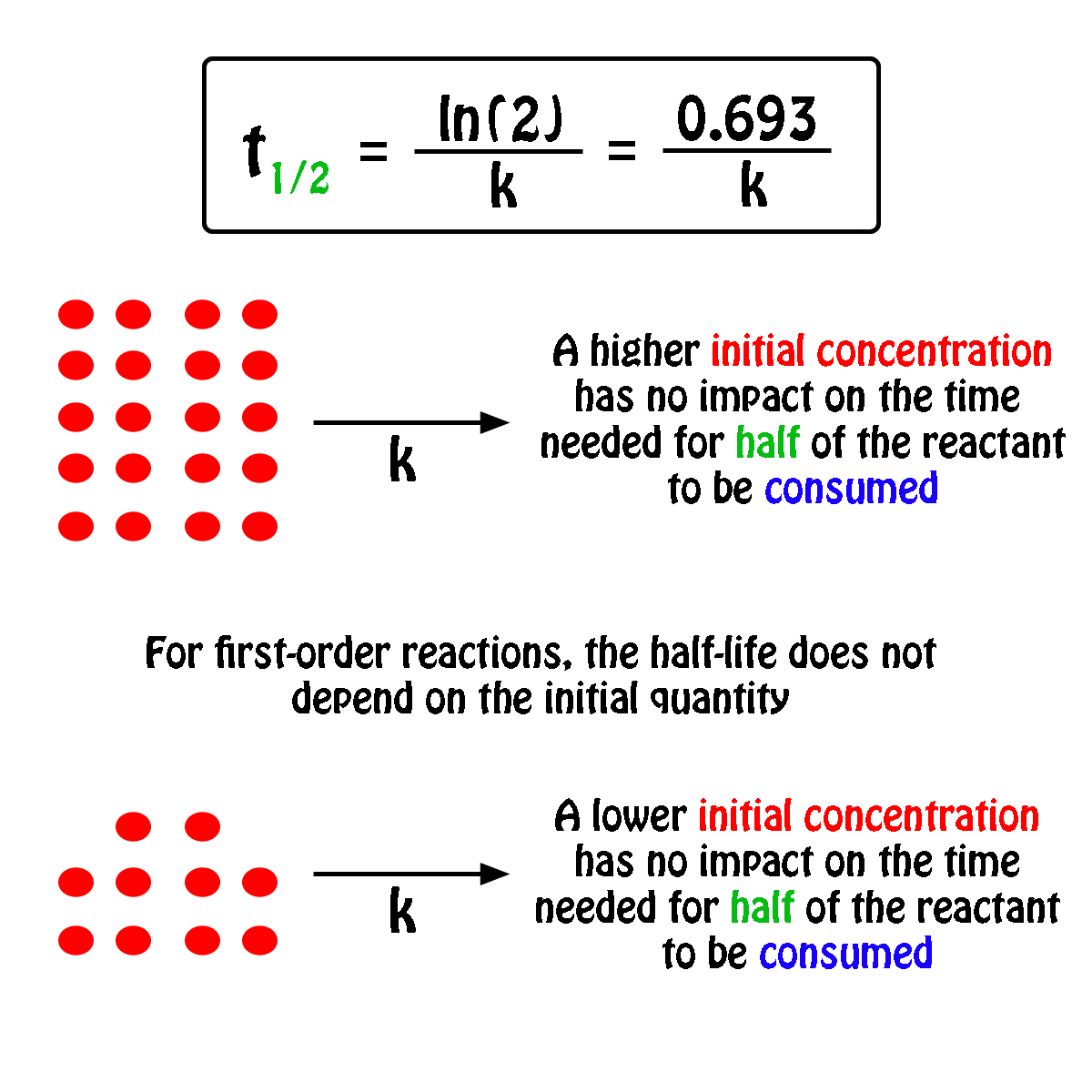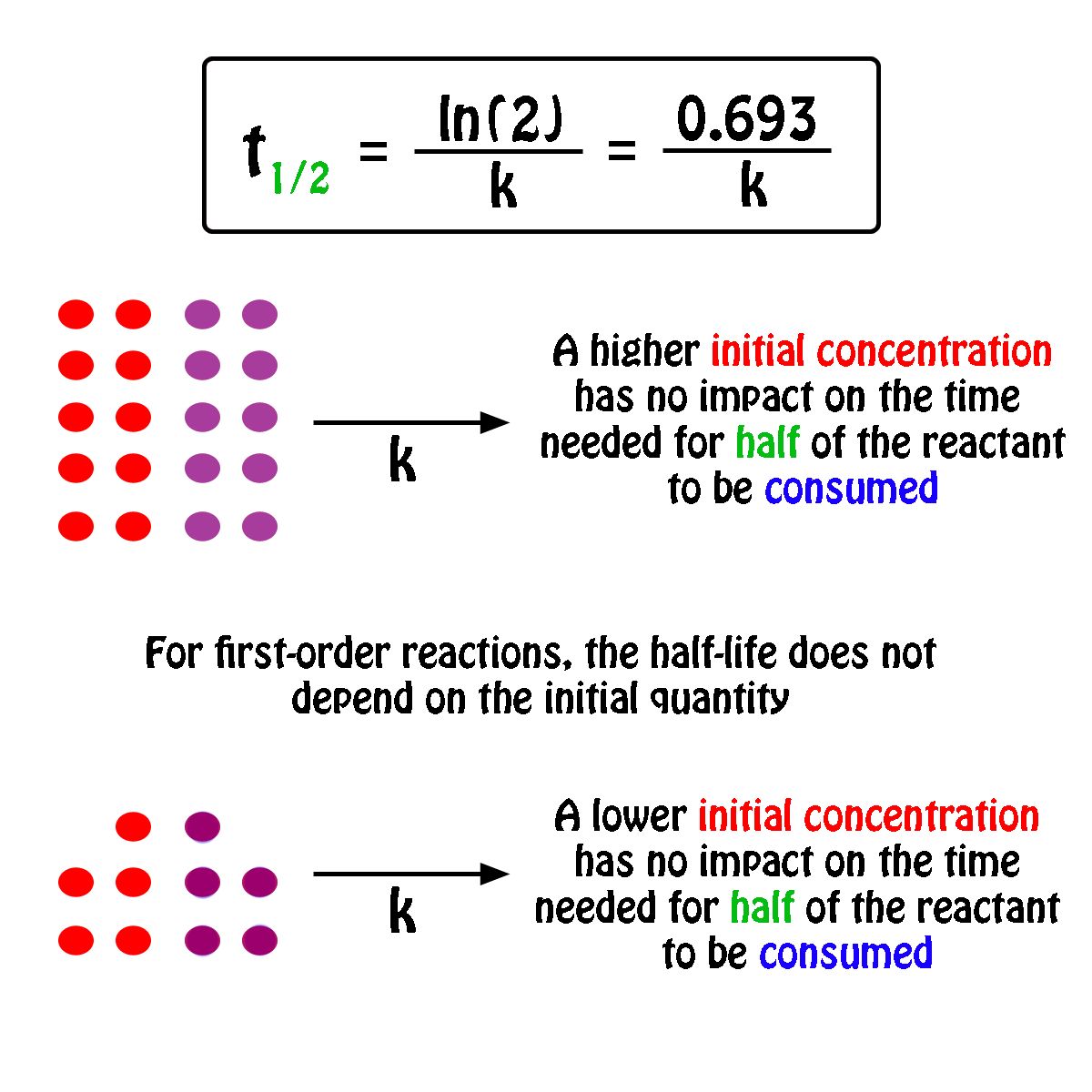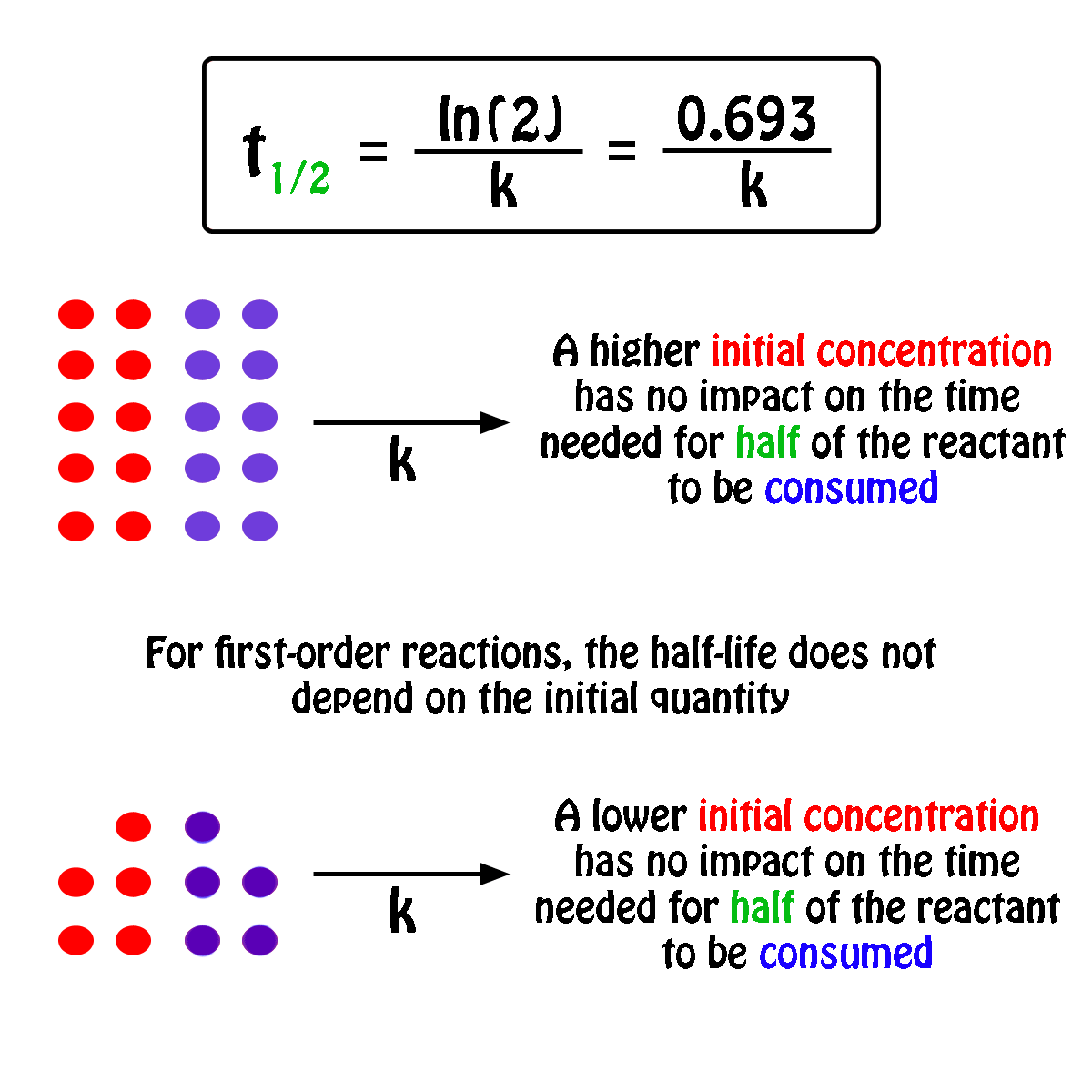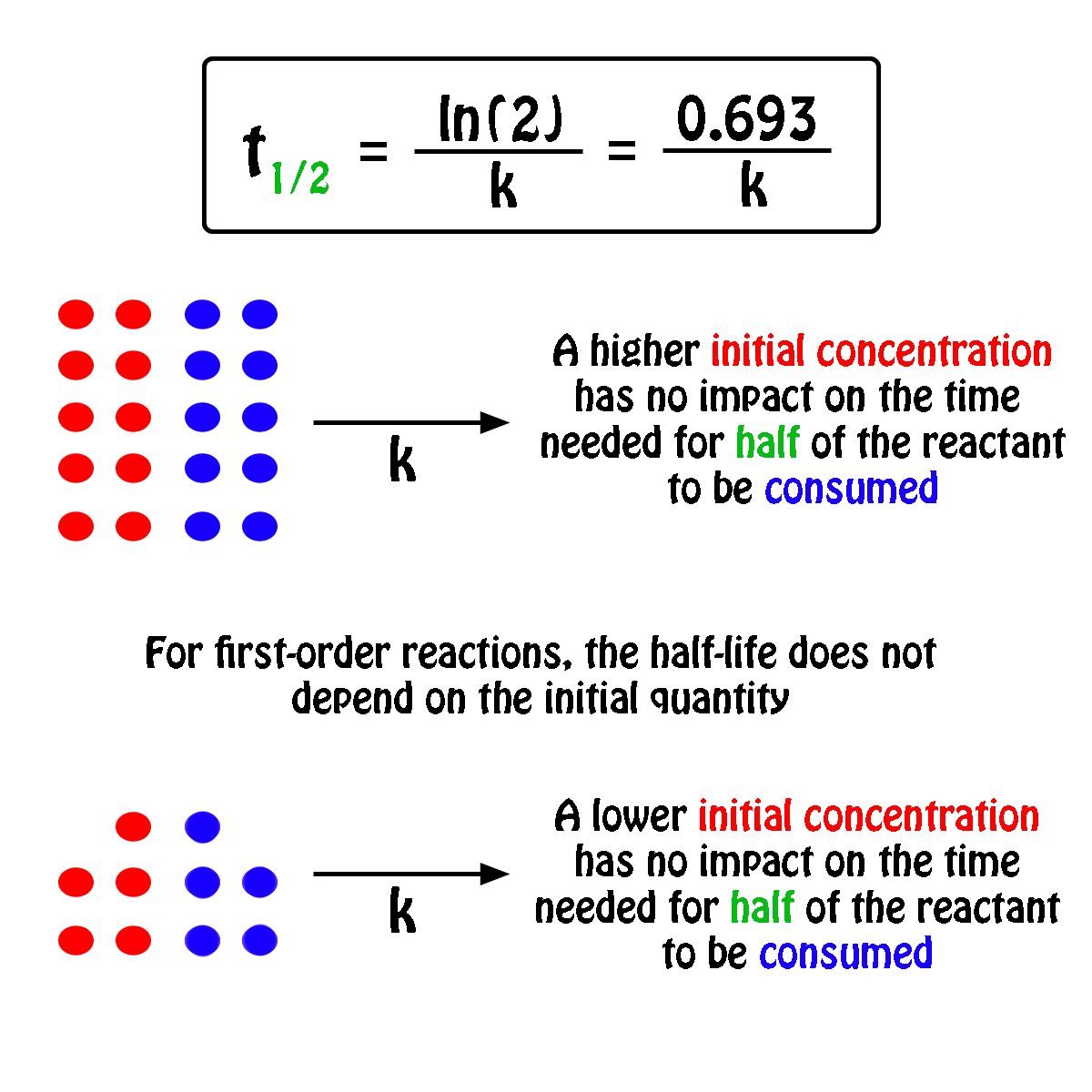
The half-life, `t_"1/2"`, of a first-order reaction is independent of the initial concentration of the reactant, which is why the problem doesn't provide you with one.

In fact, all you need to know in order to calculate the half-life of a first-order reaction is the rate constant, `k`, which in your case is equal to

`k = 7.80 * 10^(-4)"s"^(-1)`

So, the half-life of a first-order reaction can be calculated by using the equation

`color(blue)(|bar(ul(color(white)(a/a)t_"1/2" = ln(2)/kcolor(white)(a/a)|)))`

Plug in your values to find

`t_"1/2" = ln(2)/(7.80 * 10^(-4)"s"^(-1)) = color(green)(|bar(ul(color(white)(a/a)color(black)("889 s")color(white)(a/a)|)))`

The answer is rounded to three sig figs.

You can thus say that for your reaction, it takes `"889 s"` for half of the concentration of the reactant to be consumed.