How do you solve root4(x^4+1)=3x4√x4+1=3x?
3 Answers
Explanation:
or
or
or
or
or
or
or
or
Explanation:
A trick to help solve roots
Explanation:
If you are not sure how to deal with big number roots build a prime factor tree. In the question you are looking for something you can obtain a whole number 4th root from. You mat only be able to do it for part of the number leaving something behind in the root. As in this case.
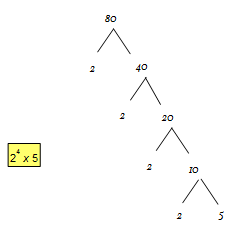 Tony B
Tony B
So


