Question

Question #35861

Answer 1

`y=(-1/20)x+-7/20`

Explanation:

The Average rate function is the straight line drawn between the points at each end of the interval.

So first we need to find our points,

`y=1/(x-2)`

`y(-3)=1/((-3)-2)`

`y(-3)=1/-5`

giving us `(-3,-1/5)`

`y(-2)=1/((-2)-2)`

`y(-2)=1/-4`

giving us `(-2,1/-4)`

So the gradient is equal to `"rise"/"run"`

rise`=-1/4-(-1/5)`

rise`=-1/20`

run`=-2-(-3)`

run`=1`

So `"rise"/"run"=(-1/20)/1=-1/20`

This is our average rate of change between the two points.

to find the function we use,

`y=mx+c`

`y=(-1/20)x+c`

And subbing in one of our points, `(-3,-1/5)`

`(-1/5)=(-1/20)(-3)+c`

`c=-7/20`

leaving us with the average rate function between `[-3,-2]` as,

`y=(-1/20)x+-7/20`

Answer 2

Average rate of change `=-(1/20)`
`x+20y+7=0`

Explanation:

`y=1/(x-2)`
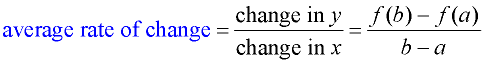
Given `a=-3, b=-2`
`f(a)=1/(-3-2)=-(1/5)`
`f(b)=1/(-2-2)=-(1/4)`

Average rate of change `=(f(b)-f(a))/(b-a)=(-(1/4)+(1/5))/((-2)-(-3)`
`=-(1/20)/1=-(1/20)`

To find the equation :
`a=x=-3,y=f(a)=-(1/5), slope = -(1/20)`

Equation is `y=mx+c`
`-(1/5)=-(1/20)(-3)+c`
`c=-(1/5)-(3/20)=-(7/20)`

`y=-(1/20)x-(7/20)`
`x+20y+7=0`