A triangle has corners at points A, B, and C. Side AB has a length of #8 #. The distance between the intersection of point A's angle bisector with side BC and point B is #4 #. If side AC has a length of #9 #, what is the length of side BC?

1 Answer
Shwetank Mauria
Dec 5, 2016

#BC=8.5#

Explanation:

Angle bisector of an angle in a triangle divides the opposite side in the ratio of its adjacent sides.
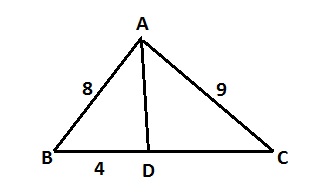
enter image source here
For example in the above diagram, angle bisector #AD# of #/_A# divides #BC# in the ratio #AB:AC# i.e.

#(BD)/(DC)=(AB)/(AC)#

In the given case, we have #AB=8#, #AC=9# and #BD=4#.

Hence #4/(DC)=8/9#

and #DC=(4xx9)/8=(cancel4xx9)/(cancel8^2)=9/2=4.5#

and #BC=4+4.5=8.5#

Related questions
See all questions in Angle Bisector Theorem
Impact of this question
1777 views around the world
You can reuse this answer
Creative Commons License