A cylindrical pillar, with a regular nonagonal cross-section, has to be carved out of a right circular cylindrical sandal wood. If the height is 10' and diameter of the base is 1', how do you prove that the minimum possible scrap is 0.623 cft, nearly?
1 Answer
Jan 7, 2017
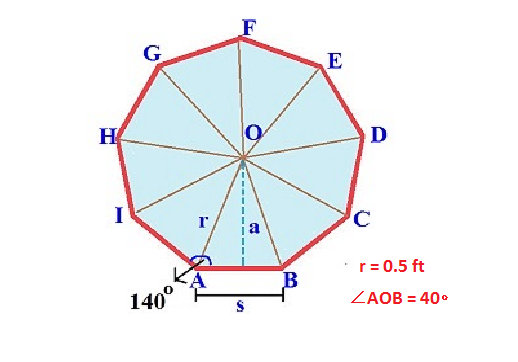
The nonagonal cross section of the pillar has been shown in the above figure. Here
So area of the nonagonal cross section is equal to the total area of 9 identical isosceles triangles each having area equal to the area of
So the nonagonal cross sectional area
Height of the pillar
So volume of the pillar of nonagonal cross section
The original volume of the cylindrical pillar
So the volume of the minimum possible scrap is

