An object with a mass of #8 kg# is on a plane with an incline of # - pi/12 #. If it takes #12 N# to start pushing the object down the plane and #5 N# to keep pushing it, what are the coefficients of static and kinetic friction?
1 Answer
the static coefficient of friction is
the kinetic coefficient of friction is
Explanation:
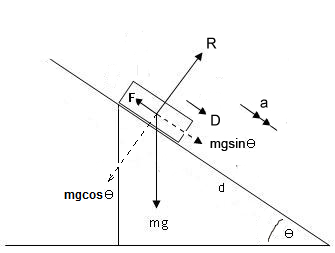
For our diagram,
If we apply Newton's Second Law up perpendicular to the plane we get:
#R-mgcostheta=0#
#:. R=8gcos(pi/12) \ \ N#
Initially it takes
# D+mgsin theta -F = 0 #
# :. F = 12+8gsin (pi/12) \ \ N#
And the friction is related to the Reaction (Normal) Force by
# F = mu R => 12+8gsin (pi/12) = mu (8gcos(pi/12)) #
# :. mu = (12+8gsin (pi/12))/(8gcos(pi/12)) #
# :. mu = 0.426409 ... #
Once the object is moving the driving force is reduced from
# D+mgsin theta -F = 0 #
# :. F = 5+8gsin (pi/12) \ \ N#
And the friction is related to the Reaction (Normal) Force by
# F = mu R => 5+8gsin (pi/12) = mu (8gcos(pi/12)) #
# :. mu = (5+8gsin (pi/12))/(8gcos(pi/12)) #
# :. mu = 0.333974 ... #
So the static coefficient of friction is
the kinetic coefficient of friction is

