Question

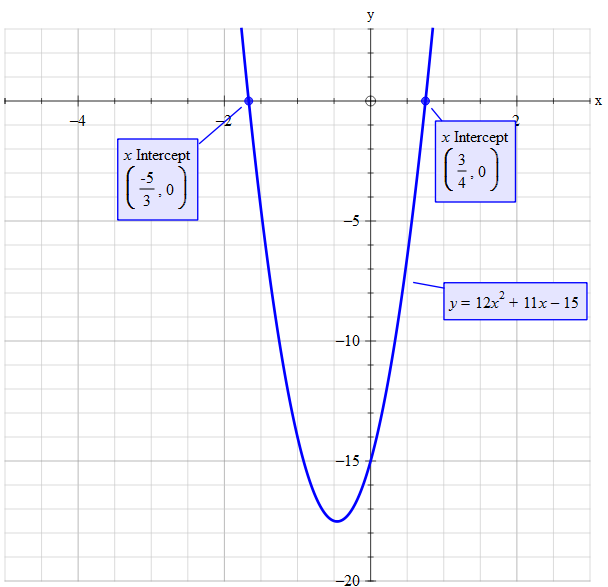
How do you solve `12x^2=-11x+15` by graphing?

Answer 1

`x=-5/3 and x =3/4`

Explanation:

First make the equation equal to zero.

`-12x^2-11x +15` = 0

Then sketch the curve

graph{-12x^2-11x+15 [-19.66, 20.34, -5.68, 14.32]}

The answers to `x` are where the curve crosses the `x`-axis.
(The equation of the `x`-axis is `y=0`)

`x=0.75 and x= -1.67`

To see if you got it right, use the quadratic formula.

Answer 2

Solution by graphing

Explanation:

Given:`" "12x^2=-11x+15`

I choose to make the `12x^2` positive so I move all the values to the right of = to the left of it.

Add `11x` to both sides

`12x^2+11x=15`

Subtract 15 from each side

`12x^2+11x-15=0`

We are instructed to solve by graphing. Unless the values are obvious you will not obtain exact values this way. The values obtained are down to how well you draw your curve and your observation of the relevant values.

You would do this:

Set `y=12x^2+11x-15`

You are particularly interested in `y=0`

Produce a table of values. For example you may choose the following set of values for `x`:

You would then draw your graph and determine the `x` values where the graph crosses the x-axis.

I am using dedicated software that actually gives the answer: