Question #65a2a
1 Answer
Apr 16, 2017
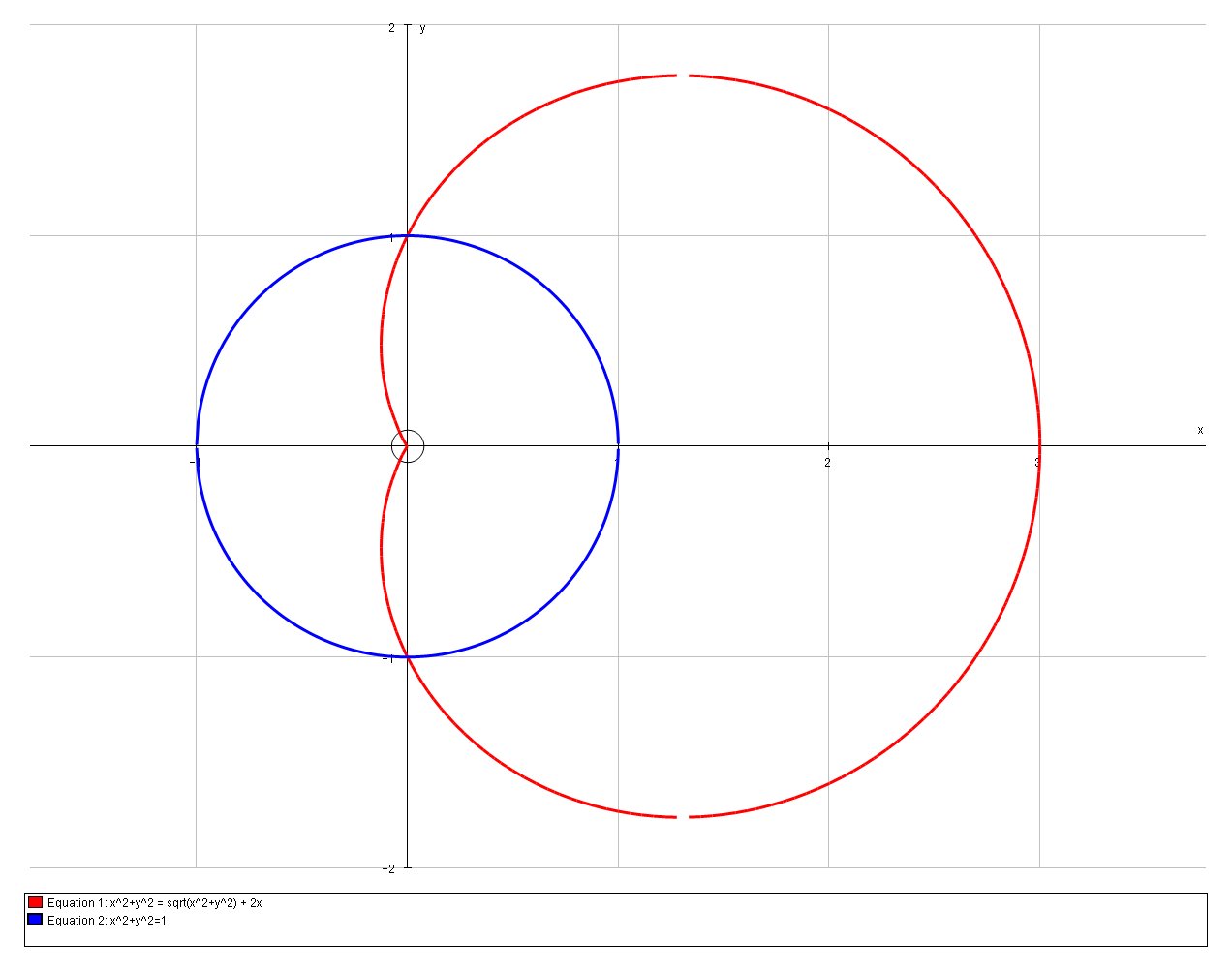
x^2+y^2 = sqrt(x^2+y^2) + 2xx2+y2=√x2+y2+2x
intersection coordinates are
Explanation:
Our Polar equation is:
r = 1+2cos thetar=1+2cosθ
To convert from polar to rectangular (Cartesian) coordinates we use the fundamental relationship:
{: (x = rcos theta), (y = rsin theta) :} \ \ and\ \ x^2+y^2 = r^2
So we can transform our equation as follows:
r = 1+2cos theta
:. r^2 = r + 2rcos theta
:. x^2+y^2 = sqrt(x^2+y^2) + 2x
For the interaction with a unit circle which would have the equation:
x^2+y^2 = 1
We would have:
:. 1 = 1 + 2x => 2x =0
" " => x=0
And:
x=0 => y^2 = 1
" " => y = +-1
So the intersection coordinates would be