Question #6074d
2 Answers
see explanation below
Explanation:
in quadrant 2, the value of #x-axis is -ve while y-axis is +ve and it hypotenuse is +ve value.
sin = y-axis/hypotenuse = +ve value.
cosec = 1/sin = hypotenuse/y-axis = +ve value.
the rest are involve with the #x-axis and become -ve value.
It has to do with the signs of the x and y axis in the second quadrant.
We let
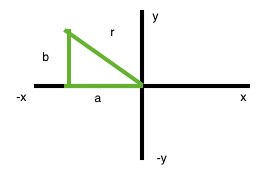
The green triangle is right because side
We have
Hopefully this helps!


