Question #f28b6
2 Answers
The height of the candle after
Explanation:
A candle is 10 in. tall after burning for 2 hours. After 3 hours, it is 8.5 in. tall. How tall will the candle be after 6 hours?
We can make a linear equation to model the situation. Since time is the independent variable and height is the dependent variable, we can let
Consider point-slope form:
where
Now, we can write a linear equation using point-slope form:
Since we want to know the height of the candle after 6 hours, we simply plug
Therefore, the height of the candle after
In support of the solution submitted by 'Y'
4 inches
Explanation:
Sometimes it is a good idea to 'scribble' a quick sketch to assist in visualisation of what the question is asking.
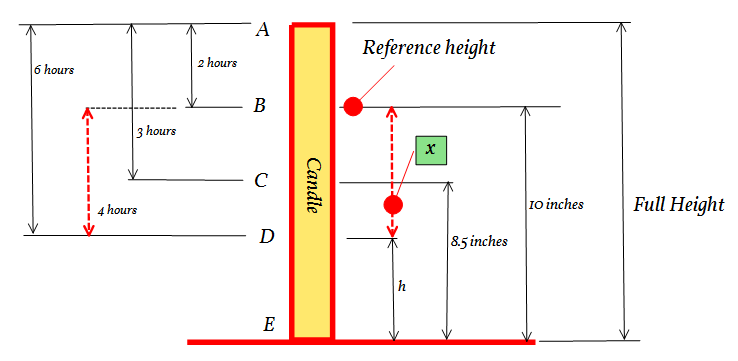 Tony B
Tony B
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Let the required height be
What the target is:
Reference height -
where
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~


