Question

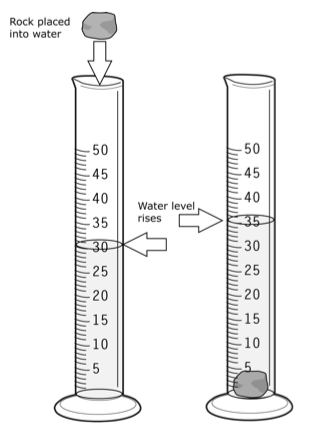
Question #945e2

Answer 1

`"1.34 g cm"^(-3)`

Explanation:

The idea here si that the volume of the rock will be equal to the volume of water it displaces when added to the graduated cylinder.

The volume of the water goes from

`"25.0 mL " -> " 36.2 mL"`

after the rock is added, so you can say that the volume of the rock will be equal to

`V_"rock" = "36.2 mL" - "25.0 mL"`

`V_"rock" = "11.2 mL"`

Now, in order to find the density of the rock, you must determine the mass of exactly one unit of volume the rock would occupy.

You know that a volume of `"11.2 mL"` corresponds to `"15.0 g"`, so you can say that `"1m L"` will correspond to

`1 color(red)(cancel(color(black)("mL"))) * "15.0 g"/(11.2color(red)(cancel(color(black)("mL")))) = "1.339 g"`

Now, you should know that

`"1 mL" = "1 cm"^3`

This means that a volume of `"1 cm"^3` corresponds to `"1.339 g"`. You can thus say that the density of the rock is equal to

`color(darkgreen)(ul(color(black)("density = 1.34 g cm"^(-3))))`

The answer is rounded to three sig figs.