How do you solve #sqrt[x+5]=3x-7# and find any extraneous solutions?
2 Answers
Explanation:
Simplifying the equation would involve first getting rid of the square root:
Simplifying further, we can expand out the square on the right side of the equation:
Since this is a quadratic equation, we want to put everything on one side and then find the zeros of the expression:
Using the quadratic formula (since we cannot factor the expression),
Simplifying gives:
Using a calculator to find the value of this expression,
Plugging the first value back into the equation, we get a valid equation:
However, when we plug the second value back into the equation, we have this:
Since the right side is negative, the second value that we got for
all values of
Explanation:
For the solution to remain within the set of numbers called Real the square root must be of a value that is not negative.
Thus all values of
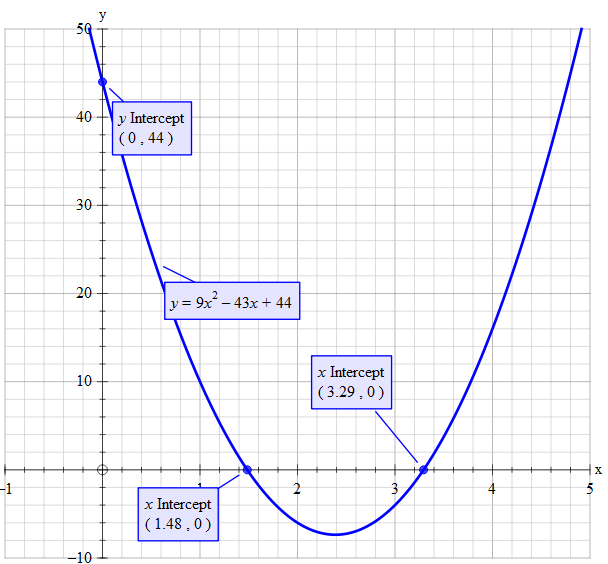
Given:
Square both sides
Subtract
To comply with convention write as:
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
Compare to
In this case:
However, as per smartspot2, substituting 1.485 back into the original question proves this value to fail if you use the 'Principle Square Root'. Thus by his approach there is only 1 value that is correct: