Question

How can we explain why electrons don't spiral into the attracting nucleus?

Answer 1

That is the "energy" of the electron. There is no opposing force.

Explanation:

Essentially, at the atomic scale, the electron is orbiting in a vacuum. With no "drag" there is nothing to reduce its angular momentum around the nucleus.

Answer 2

Here's how I would explain it.

Explanation:

This is exactly what classical physics predicts.

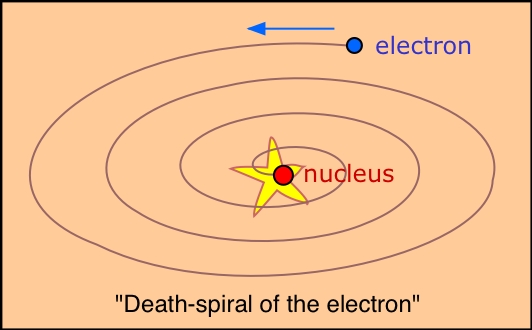
However, we now know that electrons are governed by the laws of quantum mechanics.

Electrons don't really orbit a nucleus.

Instead, we must think of the electron as a cloud of electron density.

The electron could be anywhere in a spherical "shell" around the nucleus.

We could think of the atom as consisting of many thin shells with radius `r` and thickness `Δr` arranged like the rings of an onion, as in (a) below.

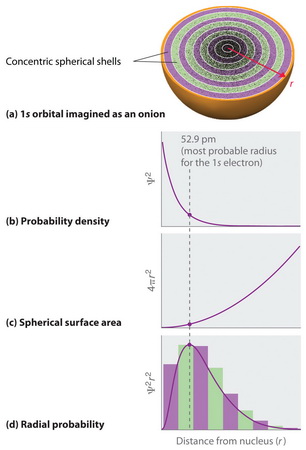
(From Chemistry LibreTexts)

For each shell, the area is `A = 4πr^2` and the volume is `V = 4πr^2Δr`.

The probability density is greatest at `r = 0` [as in (b) above].

As we move outwards, the area `A` and the volume `V` increase as in graph (c) above.

However, at large distances from the nucleus, the probability density is quite small, so the probability `P` is near zero [graph (b) above].

Also, close to the nucleus, the volume of each shell is small, so the probability `P` is near zero.

So, the highest probability of finding the electron is going to be where the volume of the shell is big enough that `P` is high, but the distance from the nucleus isn't too large for `P`to be low.

Thus, a plot of radial probability has a maximum at a certain distance from the nucleus [graph (d) above].

Most important, the probability of finding an electron at the nucleus is zero.