Question

An object with a mass of `8 kg` is on a plane with an incline of `- pi/8`. If it takes `4 N` to start pushing the object down the plane and `3 N` to keep pushing it, what are the coefficients of static and kinetic friction?

Answer 1

If angle of inclination is `(3pi)/8`:

`mu_s = 2.55`

`mu_k = 2.51`

See explanation regarding angle.

Explanation:

We're asked to find the coefficient of static friction `mu_s` and the coefficient of kinetic friction `mu_k`, with some given information.

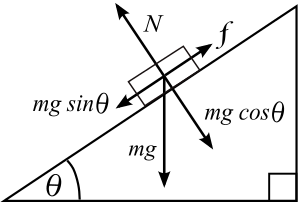
We'll call the positive `x`-direction up the incline (the direction of `f` in the image), and the positive `y` direction perpendicular to the incline plane (in the direction of `N`).

There is no net vertical force, so we'll look at the horizontal forces (we WILL use the normal force magnitude `n`, which is denoted `N` in the above image).

We're given that the object's mass is `8` `"kg"`, and the incline is `-(pi)/8`.

Since the angle is `-(pi)/8`, this would be the angle going down the incline (the topmost angle in the image above). Therefore, the actual angle of inclination is

`pi/2 - (pi)/8 = ul((3pi)/8`

The formula for the coefficient of static friction `mu_s` is

`f_s <= mu_sn`

Since the object in this problem "breaks loose" and the static friction eventually gives way, this equation is simply

`color(green)(ul(f_s = mu_sn`

Since the two vertical quantities `n` and `mgcostheta` are equal,

`n = mgcostheta = (8color(white)(l)"kg")(9.81color(white)(l)"m/s"^2)cos((3pi)/8) = color(orange)(ul(30.0color(white)(l)"N"`

Since `4` `"N"` is the "breaking point" force that causes it to move, it is this value plus `mgsintheta` that equals the upward static friction force `f_s`:

`color(green)(f_s) = mgsintheta + 4` `"N"`

`= (14color(white)(l)"kg")(9.81color(white)(l)"m/s"^2)sin((3pi)/8) + 4color(white)(l)"N" = color(green)(76.5color(white)(l)"N"`

The coefficient of static friction is thus

`mu_s = (f_s)/n = (color(green)(76.5)cancel(color(green)("N")))/(color(orange)(30.0)cancel(color(orange)("N"))) = color(red)(ulbar(|stackrel(" ")(" "2.55" ")|`

The coefficient of kinetic friction `mu_k` is given by

`color(purple)(ul(f_k = mu_kn`

It takes `3` `"N"` of applied downward force (on top of the object's weight) to keep the object accelerating constantly downward, then we have

`color(purple)(f_k) = mgsintheta + 3` `"N"`

`= 72.5color(white)(l)"N" + 3` `"N"` `= color(purple)(75.5color(white)(l)"N"`

The coefficient of kinetic friction is thus

`mu_k = (f_k)/n = (color(purple)(75.5)cancel(color(purple)("N")))/(color(orange)(30.0)cancel(color(orange)("N"))) = color(blue)(ulbar(|stackrel(" ")(" "2.51" ")|`

These values are quite high, which makes sense because the object remains in place even at a steep angle of `(3pi)/8`.