How do you solve #1/4n+12>=3/4n-4# and graph the solution on a number line?
2 Answers
Explanation:
add 4 to both sides
subtract
divide
it looks like this
your answer is
so your final equation is
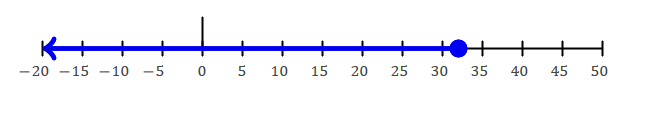
on a number line, put a closed circle on 32 and draw the line going towards the negatives indefinitely.
Here is the graph

Explanation:
Let's start by subtracting
Then, we can subtract
In order to find