Question

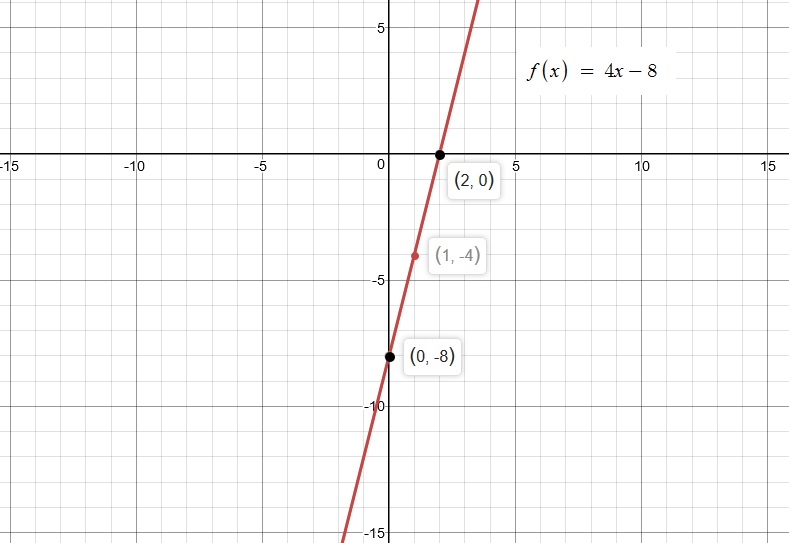
How do you graph `y=4x-8`?

Answer 1

Refer to the graph with Slope(m) = 4 and Y-Intercept `= -8`

Explanation:

`y = mx + b` is the equation of a straight line usually written this way, where `m` represents the Slope ( or Gradient ) and `b` the Y-Intercept. It is also in Slope-Intercept Form.

Also,

`m = (rise)/(run)` or ( Change in y) `/` (Change in x)

We need to graph `y = 4x - 8`

We observe that it is already in Slope-Intercept Form.

Hence, Slope = `m = 4`

Y-Intercept `= (-8)`

Go to the graph and plot the point the point `(0, -8)` on the y-axis.

Then rise 4 and run 1. Plot the point `(1, -4)`

Then again "rise 4" and then "run" 1. Plot the point `(2, 0)`

Join all these points on the graph and you will find a straight line. graph{4x - 8 [-19.75, 20.25, -14.88, 5.12]}

Also refer to an additional graph available with my suggested solution with points plotted.

Hope this helps.