Question

In a ground-state `"P"` atom in the gas phase, how many electrons have the quantum numbers `n = 3, l = 1, m_l = –1` ?

Answer 1

One electron.

Explanation:

The key here is the electron configuration of a neutral atom of phosphorus, which looks like this

`"P: " 1s^2 2s^2 2p^6 3s^2 3p^3`

Now, the `p` subshell contains a total of `3` orbitals. According to Hund's Rule, every orbital present in a given subshell must be half filled before any one of the orbitals can be completely filled.

This means that all the three `p` orbitals present in the `p` subshell must contain one electron, i.e. be half-filled, before any one of these orbitals can contain two electrons, i.e. be completely filled.

Notice that phosphorus has `3` electrons in the `3p` subshell. This tells you that each `3p` orbital will hold a single electron.

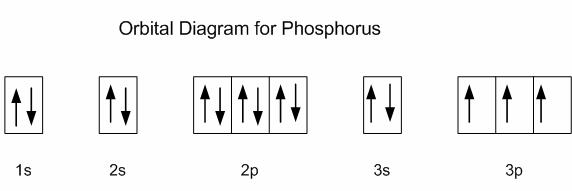
Now, you know that the principal quantum number, `n`, tells you the energy shell in which an electron is located inside an atom.

In your case, you have

`n=3 ->` the third energy shell

The angular momentum quantum number, `l`, describes the energy shell in which the electron is located.

You know that you have

• `l = 0 ->` the `s` subshell
• `l = 1 ->` the `p` subshell
• `l = 2 ->` the `d` subshell
  `vdots`

and so on. In your case, you're dealing with the `p` subshell.

Finally, the magnetic quantum number, `m_l`, tells you the orientation of the orbital in which the electron is located. For the `p` subshell, you have

`l = 1 implies m_l = {-1, 0 , +1}`

In your case, you have

`m_l = -1`

which refers to one of the three orbitals present in the `3p` subshell. Since all three `3p` orbitals contain a single electron, you can say that the incomplete quantum number set

`n = 3, l =1, m_l = -1`

describes one electron present in the third energy shell, in the `3p` subshell, in one of the three `3p` orbitals--let's say `3p_y`.