How do you solve #-(x+2)^2<=0#?

Question

1360 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-12-16T13:15:19

#x in RR#

We can do this a couple of ways:

By observation, see that the smallest value on the LH side is attained with #x=-2# (since the term inside the bracket is squared, it cannot return a negative value). And so every term coming from the squaring of the bracket will be at least 0. The negative sign, then, will make every term no bigger than 0. This means that there is no #x# that will make the LH side bigger than 0.

#:. x in RR#

#-(x+2)^2<=0#

Multiply by #-1#:

#(x+2)^2<=0#

Take the square root of both sides:

#pm(x+2)<=0#

#x<=-2#

or

#-x-2<=0#

#-x<=2#

#x>=-2#

#-2<=x<=-2, :. x in RR#

Answer 2 · 2017-12-16T15:17:26

We can validate this with a sketch:

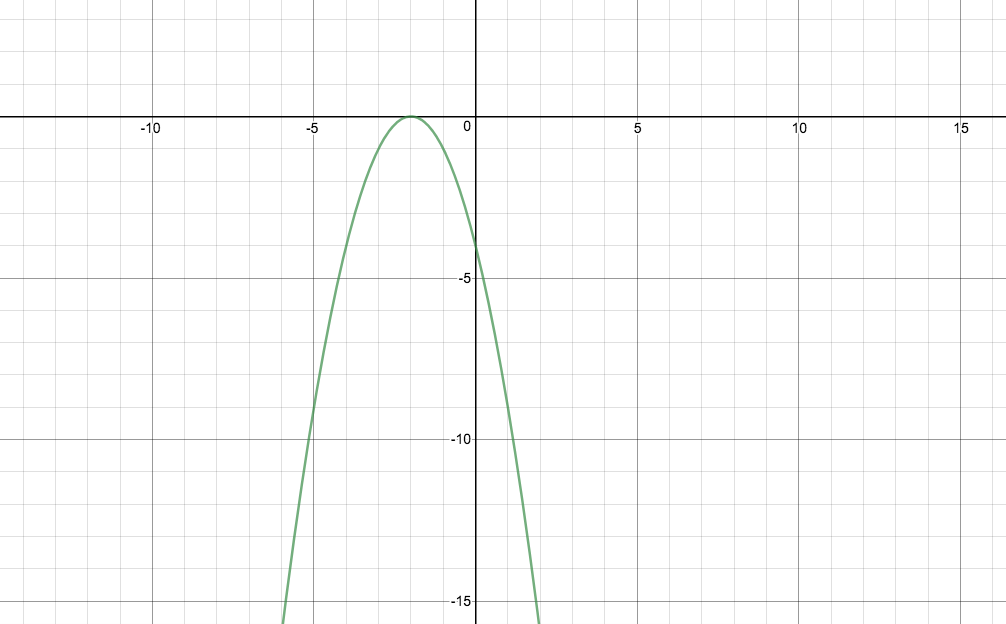
enter image source here

We see its always negative