How do I show that #f(x)=0# has one root, when #f(x)= x^3-x^2+x-6#? 4 marks
4 Answers
Explanation:
Show that
Explanation:
We know that, because of the
If
Changes from increasing to decreasing slopes imply that at any such point the slope of the function must be zero;
in this case:
The determinant of this:
however in this case, the determinant
so there is no such "turning" (aka "critical") point.
Therefore the function can only cross the X-axis once.
As a help in verification, here is a graph of the original function
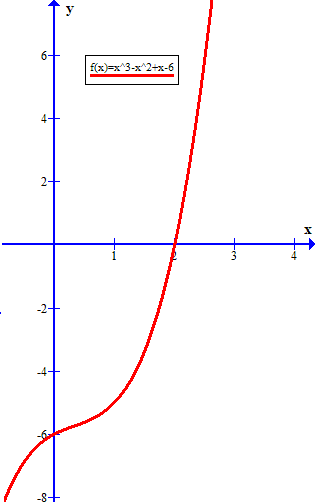
You can use the discriminant...
Explanation:
Given:
#f(x) = x^3-x^2+x-6#
The discriminant
#Delta = b^2c^2-4ac^3-4b^3d-27a^2d^2+18abcd#
In our example,
#Delta = 1-4-24-972+108 = -891#
Since
For the discriminant
#Delta > 0# means that the cubic has#3# real zeros.
#Delta = 0# means that the cubic has a repeated real zero, which may be of multiplicity#3# or#2# . If multiplicity#2# then the third zero is also real.
#Delta < 0# means that the cubic has one real zero and a complex conjugate pair of non-real zeros.
There are several ways to answer this question. Concentrate on the one that looks most like what you've been studying.
Explanation:
Use the Intermediate Value Theorem to show that there is at least one root.
Use Rolle's or the Mean Value Theorem to show that there cannot be two roots.
Conclude that there is exactly one root.



